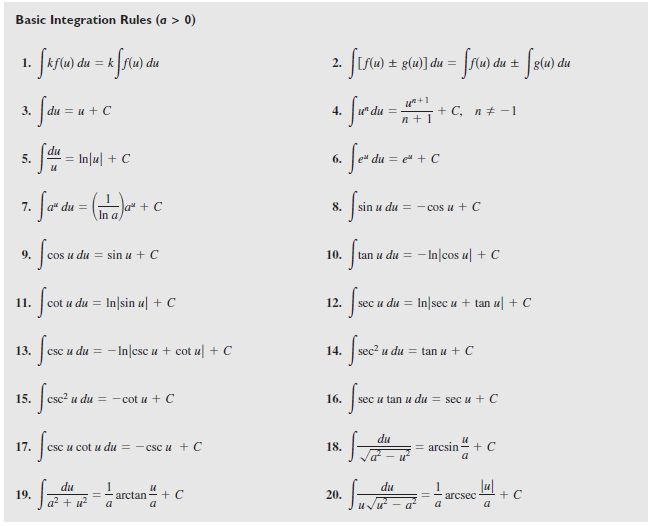
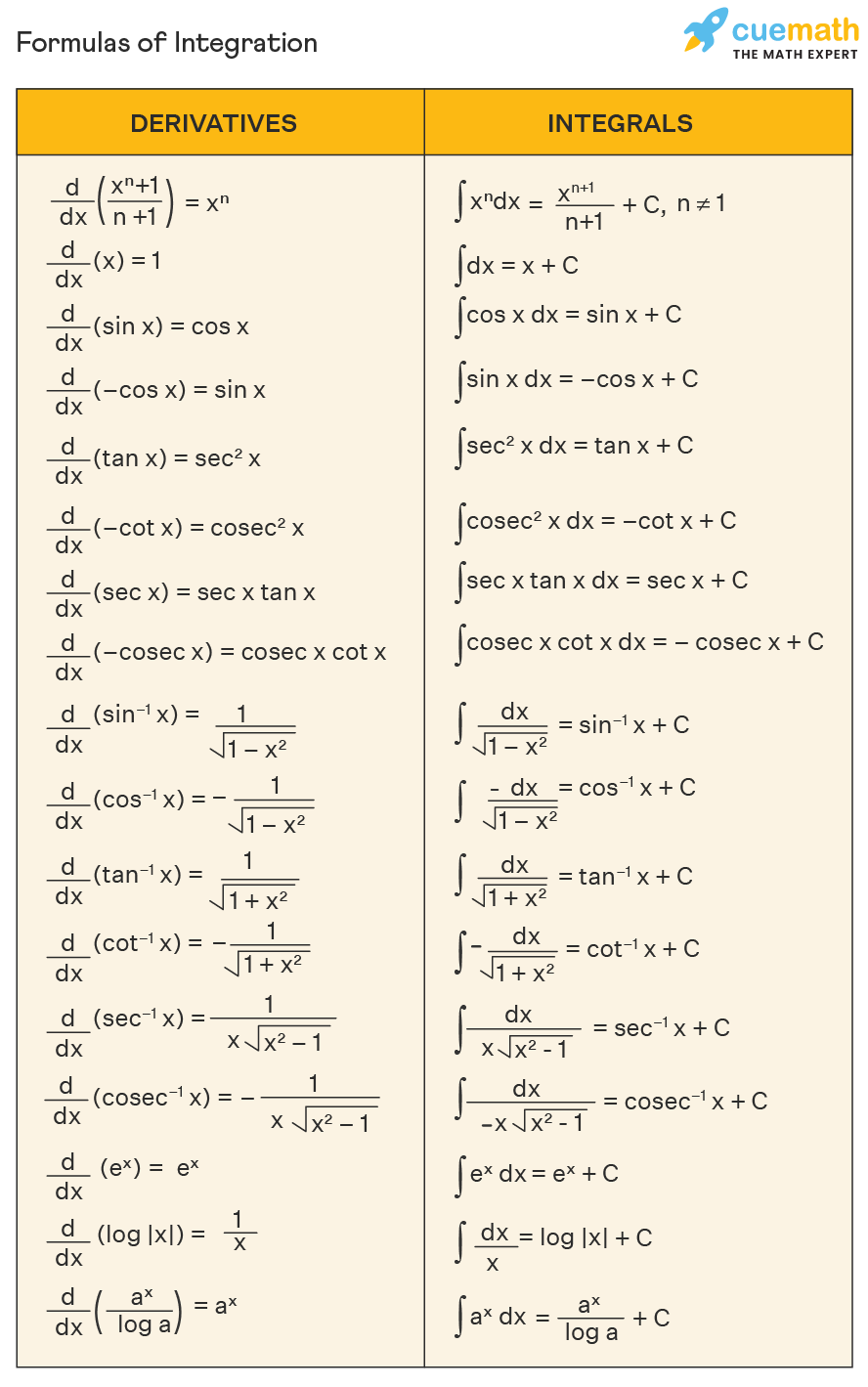
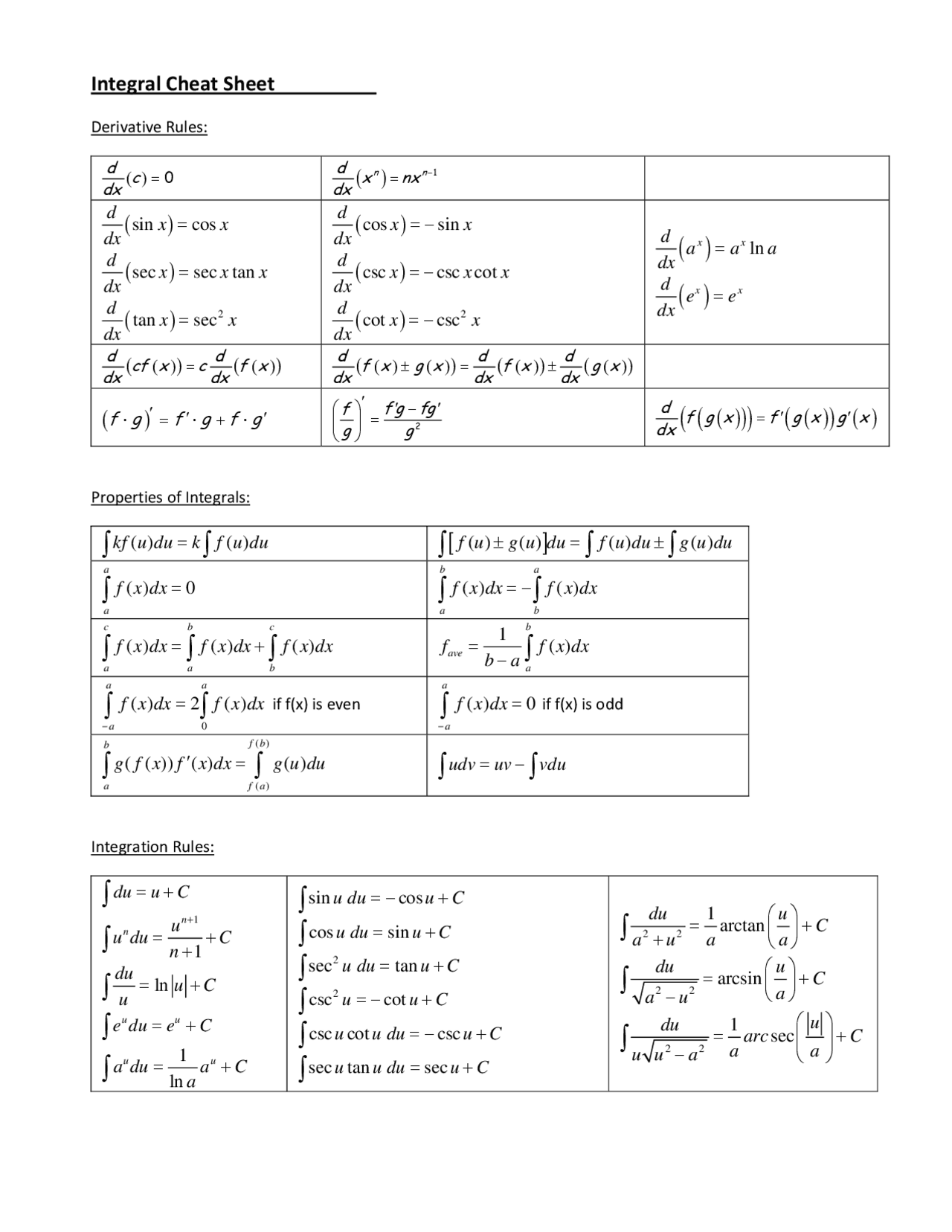
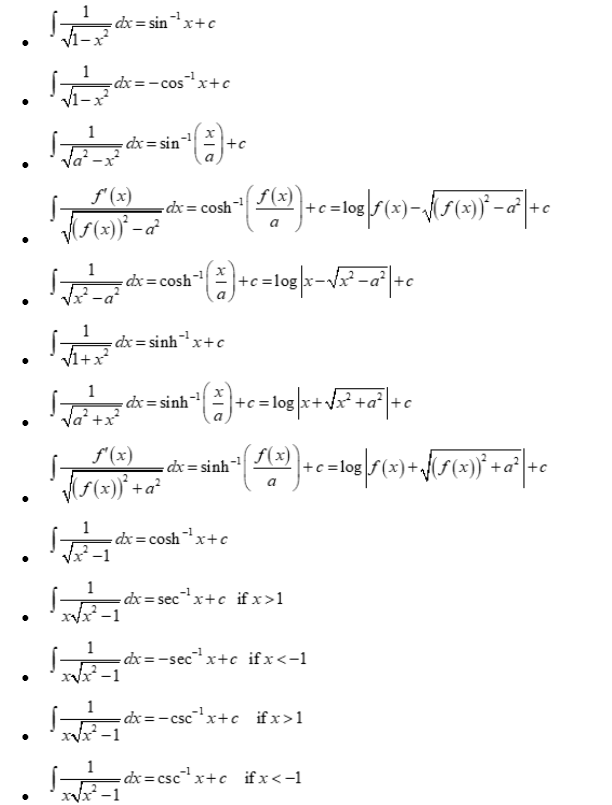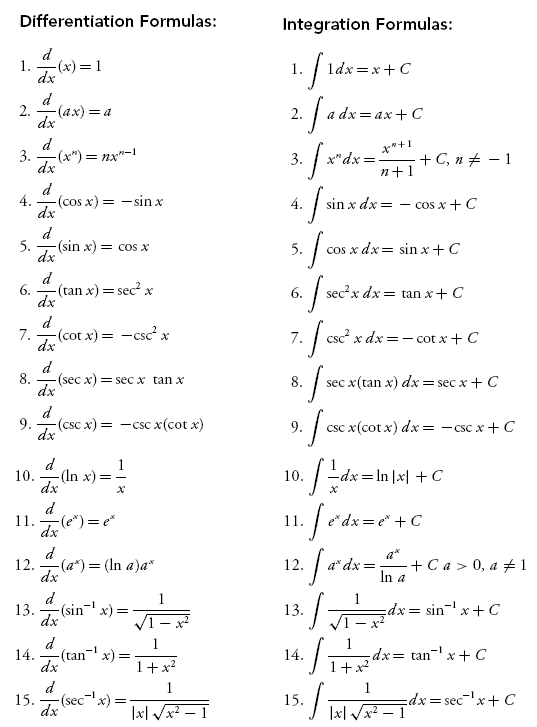Integral Rules Sheet

Integral Rules Sheet - ′= −∫ ′ ∫integral of a constant: Cheat sheet for integrals 1. Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. ( ) 𝑥=𝑥⋅ ( ) ∫taking a constant out: ⋅ (𝑥 ) 𝑥= ⋅∫ 𝑥 𝑥 ∫sum/difference. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2;

Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. ( ) 𝑥=𝑥⋅ ( ) ∫taking a constant out: ⋅ (𝑥 ) 𝑥= ⋅∫ 𝑥 𝑥 ∫sum/difference. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx. ′= −∫ ′ ∫integral of a constant: Cheat sheet for integrals 1. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2;

Cheat sheet for integrals 1. ( ) 𝑥=𝑥⋅ ( ) ∫taking a constant out: Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2; Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. ⋅ (𝑥 ) 𝑥= ⋅∫ 𝑥 𝑥 ∫sum/difference. ′= −∫ ′ ∫integral of a constant: Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx.

Derivative Rules Cheat Sheet

′= −∫ ′ ∫integral of a constant: Cheat sheet for integrals 1. Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx. Integrals with trigonometric functions z sinaxdx= 1 a.

Printable Integrals Table

( ) 𝑥=𝑥⋅ ( ) ∫taking a constant out: Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx. Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z.

Solved Determine which of the integrals can be found using

′= −∫ ′ ∫integral of a constant: Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2; ⋅ (𝑥 ) 𝑥= ⋅∫ 𝑥 𝑥 ∫sum/difference. Cheat.

Basic Integral Rules

Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx. Cheat sheet for integrals 1. ′= −∫ ′ ∫integral of a constant: Integrals with trigonometric functions z sinaxdx= 1 a.

Basic Rules Of Integration

′= −∫ ′ ∫integral of a constant: Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. ( ) 𝑥=𝑥⋅ ( ) ∫taking a constant out: Cheat sheet for integrals 1. ⋅ (𝑥 ) 𝑥= ⋅∫ 𝑥 𝑥 ∫sum/difference.

Page 1 of 2 Some Important Rules Of Differential & Integral Calculus

Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx. Cheat sheet for integrals 1. ( ) 𝑥=𝑥⋅ ( ) ∫taking a constant out: Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. ⋅ (𝑥 ) 𝑥= ⋅∫ 𝑥.

Basic Integral Formulas

Cheat sheet for integrals 1. ( ) 𝑥=𝑥⋅ ( ) ∫taking a constant out: ′= −∫ ′ ∫integral of a constant: ⋅ (𝑥 ) 𝑥= ⋅∫ 𝑥 𝑥 ∫sum/difference. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2;

Integrals ONE GREAT WORLD FOR ALL

Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2; ( ) 𝑥=𝑥⋅ ( ) ∫taking a constant.

Basic Rules Of Integration

′= −∫ ′ ∫integral of a constant: ( ) 𝑥=𝑥⋅ ( ) ∫taking a constant out: Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. Cheat sheet for integrals 1. ⋅ (𝑥 ) 𝑥= ⋅∫ 𝑥 𝑥 ∫sum/difference.

Integral cheat sheet Docsity

′= −∫ ′ ∫integral of a constant: Integral of a constant \int f\left(a\right)dx=x\cdot f\left(a\right) take the constant out \int a\cdot f\left(x\right)dx=a\cdot \int f\left(x\right)dx. ⋅ (𝑥 ) 𝑥= ⋅∫ 𝑥 𝑥 ∫sum/difference. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2; (.

Integral Of A Constant \Int F\Left(A\Right)Dx=X\Cdot F\Left(A\Right) Take The Constant Out \Int A\Cdot F\Left(X\Right)Dx=A\Cdot \Int F\Left(X\Right)Dx.

Cheat sheet for integrals 1. Integral is called convergent if the limit exists and has a finite value and divergent if the limit doesn’t exist or has infinite value. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2; ( ) 𝑥=𝑥⋅ ( ) ∫taking a constant out: